As any experimental physicist can tell you, when it comes to the measurement of any quantity, what is as important as the measurement itself, is the estimate of the error associated with the measurement. So in simple terms, if you measure the height of a building as 34.5 metres, it is as important to know whether the error in the measurement is plus or minus 20 meters or plus or minus 0.2meters. The importance of knowing the errors in any measurement is not just critical for pilots of low flying planes; it has a fundamental importance in the management of financial assets and liabilities. Many of the current ills of the financial marketplace can be placed at the doors of the mathematicians who tried to apply mathematical rigour to the financial world assuming that the same success could be achieved as that seen in the physical world in the utilisation of mathematical models to describe the world outside the lecture theatre. - The errors of this have been described very well in books such as the Black Swan by Nassim Nicholas Taleb. But perhaps it is time for the mathematicians to move aside and let the experimental physicists take the lead in applying mathematics in an imperfect world! The reason for this is quite simply, that whilst experimental physicist can claim to have a deep knowledge of mathematics, much of the application of mathematical techniques to finance has been undertaken by mathematicians, for whom the whole idea of error margins appears to be an alien concept. The effects of the lack of appreciation of error margins can be seen throughout the financial world, and arguably has been a contributing factor to the crisis in credit valuations that has led to the economic malaise the world is now in.
For pension funds, the effects of this can be seen in the way that an experimental physicist and a mathematician would approach a typical problem. A physicist, when asked to calculate the value of a set of pension liabilities would answer something like $1,500m, plus or minus $100m reflecting factors such as the errors in mortality assumptions and uncertainties in the appropriate risk free government bond yield to use when bond yields may be currently subjected to short term technical factors that have no long term significance. Moreover, this figure and the error associated with it, are likely to remain relatively stable. The figure together with the error associated with it, should be the basis on which asset allocation and any hedging decisions should be made. On this basis, a physicist may see a perfectly good rationale for having a reasonable exposure to equities if the error margins in valuations are so large that the volatility in marked-to-market equity valuations may conceivably be not vastly different in size. The error margins in the valuations means that there can be no absolutely exact matching of liabilities by government bonds, since the value of the liabilities themselves cannot be calculated beyond a certain level of precision.
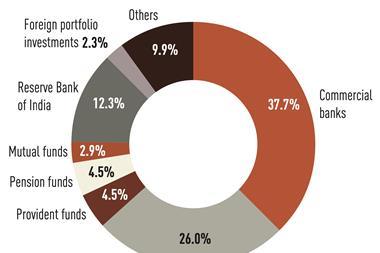
A mathematician on the other hand, may state that the liabilities are precisely $1,543.456 m based on his liability models and government bond yield curves and this value changes by the minute in the light of market movements in government bonds. Asset allocation decisions, he would argue, should be based on this and continuously rebalanced. As a result, this would lead to matching by expensively priced long dated bonds and swaps, with regular rebalancing to give the illusion of having maintained a close matching. The impact of this reasoning has led to huge demand in markets such as the UK for long dated government bonds to match liabilities, despite the fact that not only are government bond yields currently extremely low, most commentators would argue that the only way they are likely to move is upwards. Pension funds who believe they have matched their liabilities in this way, are likely to see large real losses on their bond portfolios, which are only offset by theoretical reductions in the value of pension liabilities due to the higher discount rates implied by higher government bond yields.
The economic reality is that there are large uncertainties in a valuation due both to inherent uncertainties in the maturity profile etc of the pension liabilities, and also in the actual future risk free bond yields that are the basis for any form of discounting. Current government bond yields clearly do not represent an unbiased estimate for future government bond returns with both the effects of quantitative easing as well as the artificial demand stimulated by the effect of rigid LDI approaches to matching. Using government bond yields to effectively discount pension fund liabilities may be useful for accountants and as a shorthand for estimating pension fund liabilities, but the calculated discounted value of the liabilities represents an estimate, not a true economic figure that asset allocation decisions should be based. The reality is that any economic valuation of a pension fund’s liabilities has an error margin built into it. The size of the error margin is of critical importance, since it effectively determines whether expensive approaches to matching liabilities make any sense at all. If error margins in liabilities are large, then adopting an approach of approximate matching using asset classes such as equities and other assets aimed at producing long term high absolute returns with given levels of risk may be more sensible than investing in bonds when the downside looks far more likely than the upside.
Unless there is a proper appreciation of error margins in the valuations of both assets and liabilities, pensions funds may be like the pilot of the low flying plane at night who has been given some measurements of the buildings he is flying over, but no appreciation of the error margins in the heights.










No comments yet